
Pedal
Triangles and Simson Lines
By
Tonya
DeGeorge
Pedal triangles are formed constructing perpendiculars of each side of the triangle to an arbitrary point in the plane. For instance, given the triangle ABC, we can see the Pedal triangle (in grey) that is formed in the following diagram:
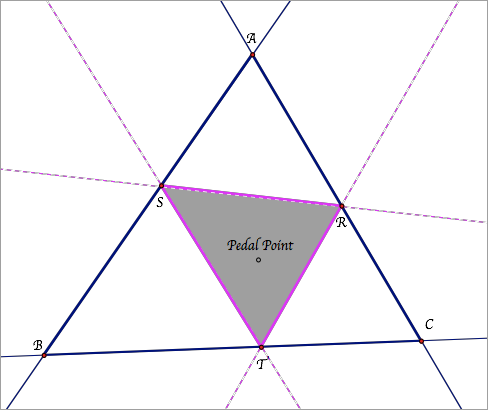
As we move the Pedal point, the pedal triangle moves as well. It can move within the triangle or even outside the triangle as well. It is also important to note that the Pedal point does not necessarily have to be inside the Pedal triangle. It can be outside the triangle, as shown below.
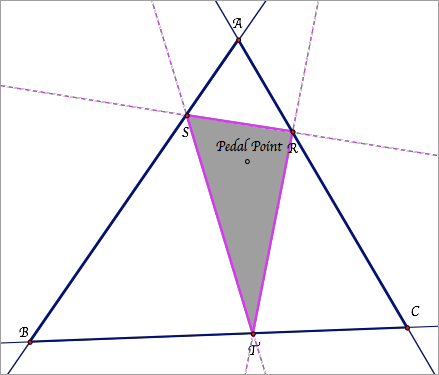
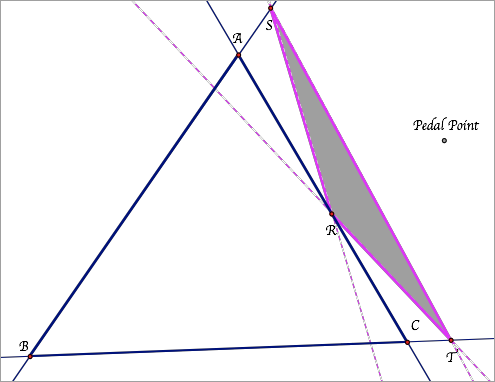
However, when moving the Pedal point around, there are times when the Pedal triangle disappears! In its place is a line segment, where all three vertices of the Pedal triangle are collinear. This line segment is called the Simson line. So when does this occur and is there a pattern for when this happens?
LetŐs begin the investigation by looking at areas where this occurs. By moving the Pedal point, we can see that the Simson line appears when the Pedal point is Ňon top ofÓ the vertices of the triangle. (The three diagrams below show the Simson line that is formed at each case: when the Pedal point is on vertex A, when the Pedal point is on vertex B, and when the Pedal point is on vertex C).
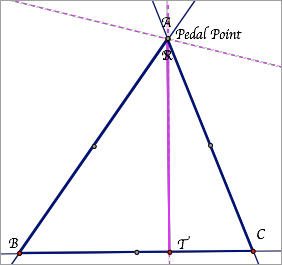

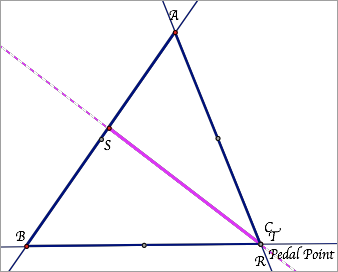

So,
normally, this would lead one to think that the Simson line appears along the
perimeter of the triangle.
However, when the pedal point is placed along one of the sides of the
triangle, this does not happen (as shown to the left)!
So
are the vertices of triangle ABC the only places where the Simson line appears? By moving the Pedal point all around
the interior of the triangle results with the Pedal triangle. Regardless of where the point is
placed, a pedal triangle is formed.
This may lead one to think that the three placements (on the vertices of
the triangle ABC) are the only locations where Simson lines form.
However, upon more investigations, we can see that this is not the case. The Simson line shows up in a number of places – when the Pedal point is outside the triangle. However, this does not mean that the Simson line appears whenever the Pedal point is outside the triangle. Again, there are certain locations where this occurs.
The following are some cases where the Simson line appears – where the Pedal point is placed at different locations.
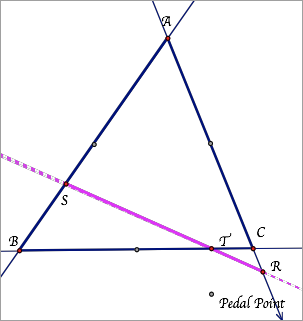
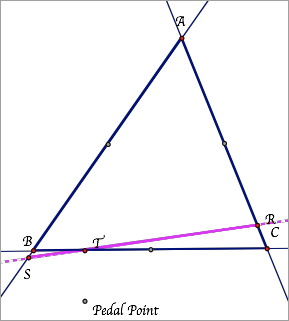
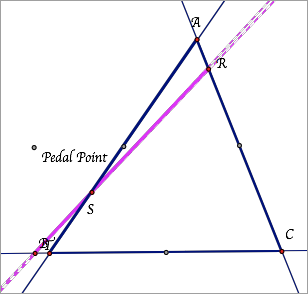


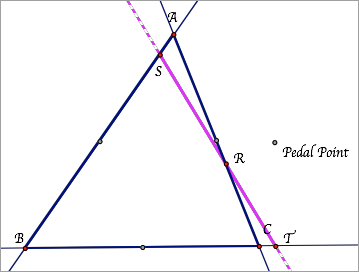
From looking at the series of pictures above it seems like the Simson line is formed when the Pedal point is on this invisible circle. But where exactly is this circle? Well, it appears that the circle the Pedal point must move along on must contain the three vertices of the triangle ABC. The circumcircle is a circle that encompasses the entire triangle and contains the vertices of the triangle on the boundary of the circle. LetŐs test this out.
If we construct the circumcircle around triangle ABC, we get:
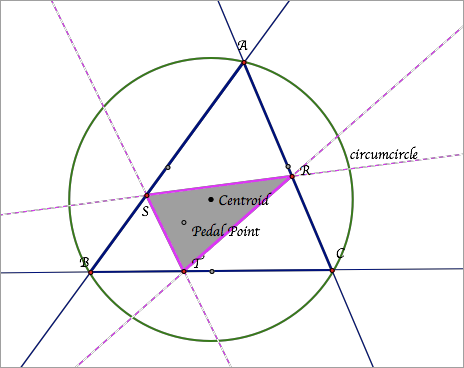
Now if we place the Pedal point on any point on the circle, we should expect to see a Simson line. And sure enough we do! We can see this in the diagram below:
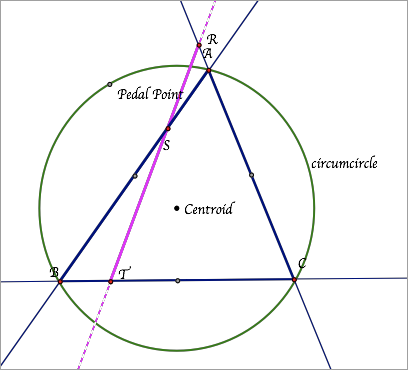
As the Pedal point moves along the circumcircle, the Simson line appears to be moving as well. Click here to see animation in GSP.
In conclusion, we can say that the Simson line is formed when the Pedal point is a point on the circumcircle of the triangle, which also includes the vertices of triangle ABC.